What is a Prime Number?
Table of Contents
Introduction
Prime Numbers
Prime numbers are a fascinating aspect of mathematics that have intrigued scholars and mathematicians for centuries. In this article, we will delve into the world of prime numbers, uncovering their properties, understanding how to find them, and distinguishing them from composite numbers.
Analogy of Definition
What is a Prime Number?
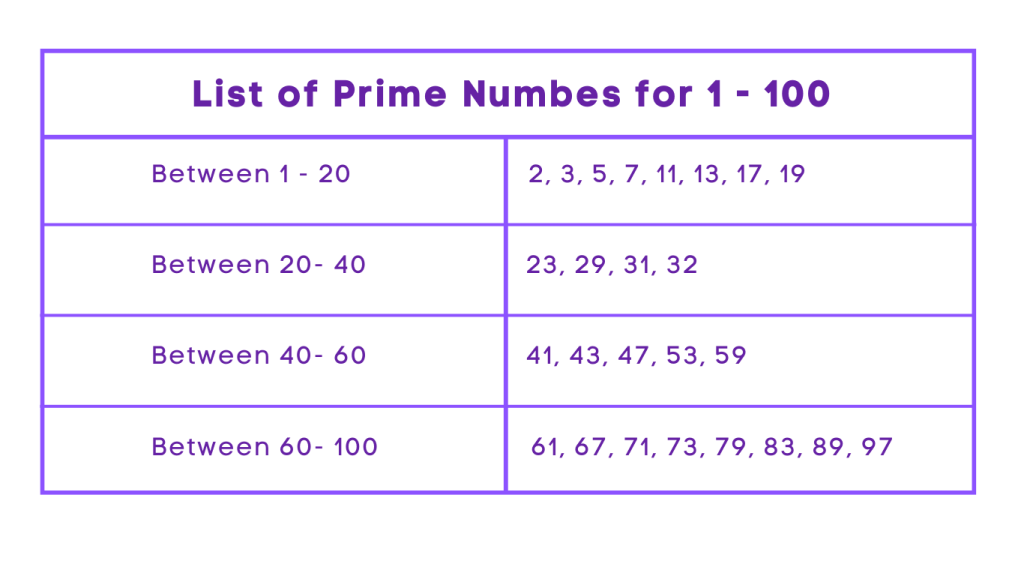
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. In simpler terms, it is a number that is only divisible by 1 and the number itself. For example, 2, 3, 5, 7, 11, and 13 are all prime numbers. There are in total 25 prime numbers from number 1 to 25. The list of prime number includes , 3, 5, 7, 11, 13, 17, 19, 23, 29, 31, 37, 41, 43, 47, 53, 59, 61, 67, 71, 73, 79, 83, 89, 97.
Properties of Prime Numbers
– Prime numbers are only divisible by 1 and themselves.
– The number 2 is the only even prime number.
– There are infinitely many prime numbers.
– Every composite number can be expressed as a unique product of prime numbers, known as the Fundamental Theorem of Arithmetic.
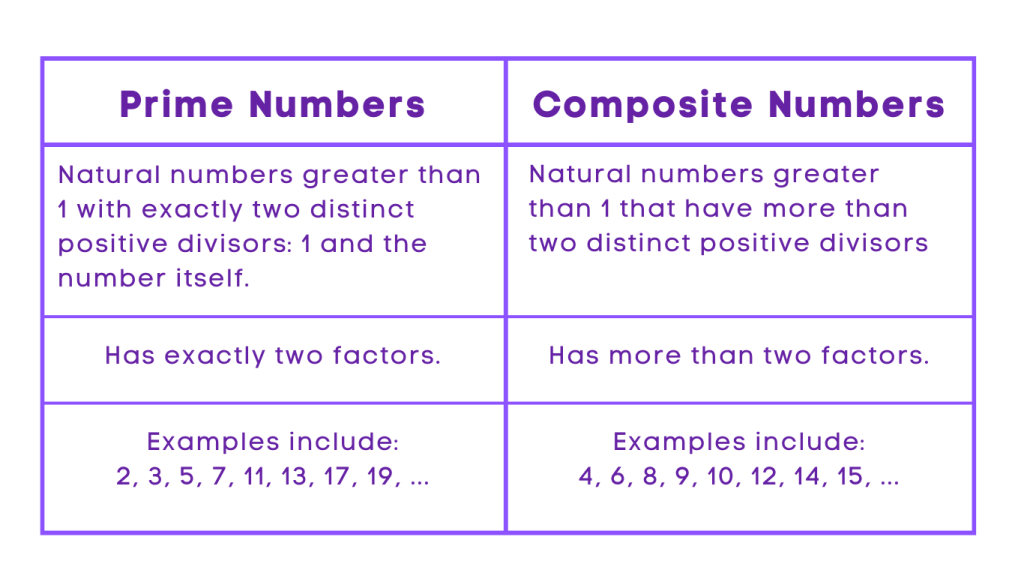
Difference Between Prime and Composite Numbers
The key differences between prime and composite numbers are:
– Prime numbers have exactly two distinct positive divisors, while composite numbers have more than two distinct positive divisors.
– The number 1 is neither prime nor composite.
– Prime numbers are the building blocks of composite numbers, as every composite number can be expressed as a unique product of prime numbers.
Method
How to Find Prime Numbers?
Finding prime numbers is a fascinating mathematical endeavor that can be approached with a straightforward method. Here’s a step-by-step guide to identify prime numbers:
Step 1: tart with the number you want to check.
Step 2: Begin by dividing the number by 2, since any even number greater than 2 cannot be prime. If the number is divisible by 2, then it’s not a prime number. If it’s not divisible by 2, proceed to the next step.
Step 3: Next, check divisibility by odd numbers starting from 3. Divide the number by 3, then by 5, then by 7, and so on, up to the square root of the number being tested.
Step 4: If the number is not divisible evenly by any of these smaller numbers, then it is a prime number.
For example, let’s find out if 13 is a prime number:
Start with 13.
Check divisibility by 2 (even) – it’s not divisible.
Check divisibility by odd numbers: 3, 5, 7, 9, 11.
None of these numbers divide evenly into 13.
So, 13 is a prime number.
This method helps identify prime numbers efficiently, making it accessible even for those new to mathematics.
Using Formula to Find Prime Numbers
You can use the formula ‘6n + 1’ or ‘6n – 1’ to find prime numbers efficiently. Here’s how it works:
- Start with a value of n: Begin by selecting a value for ‘n’. This value can start from 1 and increase gradually.
- Calculate 6n + 1 and 6n – 1: Plug the chosen value of ‘n’ into the formulas ‘6n + 1’ and ‘6n – 1’ to generate two numbers.
- Check for divisibility: Test whether the numbers generated by the formulas are prime by dividing them only by prime numbers less than the square root of the generated number. If neither of them is divisible by any smaller prime numbers, then they are prime numbers themselves.
For example, let’s find prime numbers using this method:
- Start with n = 1:
- 6n + 1 = 6(1) + 1 = 7
- 6n – 1 = 6(1) – 1 = 5
- Both 7 and 5 are prime numbers.
- Increase n:
- For n = 2:
- 6n + 1 = 6(2) + 1 = 13
- 6n – 1 = 6(2) – 1 = 11
- Both 13 and 11 are prime numbers.
- For n = 2:
This formula can efficiently generate prime numbers, particularly for numbers greater than 5, as it exploits the properties of divisibility by 6.
Examples
Example 1: Let’s find out if 17 is a prime number
Start with 17.
Check divisibility by 2 (even) – it’s not divisible.
Check divisibility by odd numbers: 3, 5, 7, 9, 11, 13.
None of these numbers divide evenly into 17.
So, 17 is a prime number.
Example 2: Let’s find out if 31 is a prime number:
Start with 31.
Check divisibility by 2 (even) – it’s not divisible.
Check divisibility by odd numbers: 3, 5, 7, 9, 11, 13, 15, 17, 19, 21, 23, 25, 27, 29.
None of these numbers divide evenly into 31.
So, 31 is a prime number.
Quiz
Tips and Tricks
1.Identify Prime Numbers
Tip: To identify prime numbers, start with 2 and check divisibility by integers up to the square root of the number. If it’s not divisible by any smaller number, it’s prime.
Smallest Prime Number:
2. Smallest Prime Number
Tip: The smallest prime number is 2. It stands alone as the only even prime number, as all others divisible by 2 are not prime.
Even Prime Numbers:
3. Even Prime Number
Tip:Despite the common perception, the only even prime number is 2. All other prime numbers are odd.
4, Prime Factorization
Tip: Prime factorization breaks down a number into its prime factors. This method helps identify the prime factors of composite numbers and simplifies calculations.
Real life application
Story: “The Prime Number Quest”
In a world filled with mystery and intrigue, a group of adventurers embarked on a quest to unlock the secrets of prime numbers and their real-life applications.
Challenge 1: The Cryptic Code
The adventurers encountered a cryptic code that required them to identify the prime factors of a large composite number. By applying the concept of prime factorization, they successfully decoded the message and uncovered a hidden treasure map.
Challenge 2: The Secure Vault
As they journeyed further, the adventurers encountered a secure vault protected by a series of prime number locks. By identifying the prime numbers and their properties, they were able to unlock the vault and reveal ancient artifacts of great value.
Challenge 3: The Enchanted Portal
In their final challenge, the adventurers discovered an enchanted portal that required them to find the prime numbers within a specific range to activate its magic. By using the Sieve of Eratosthenes, they systematically identified the prime numbers and gained access to a realm of untold wonders.
FAQ's
Prime numbers have exactly two distinct positive divisors, are only divisible by 1 and themselves, and are the building blocks of composite numbers.
No, 1 is not considered a prime number. By definition, a prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. Since 1 has only one positive divisor (itself), it does not meet the criteria to be classified as a prime number.
Yes, 29 is a prime number. A prime number is defined as a natural number greater than 1 that has only two positive divisors: 1 and itself. Since 29 has no other positive divisors besides 1 and 29, it meets the criteria to be classified as a prime number.
Yes, 2 is a prime number. A prime number is defined as a natural number greater than 1 that has only two positive divisors: 1 and itself. Since 2 has no other positive divisors besides 1 and 2, it meets the criteria to be classified as a prime number.
Prime numbers have exactly two distinct positive divisors, while composite numbers have more than two distinct positive divisors. Prime numbers are the building blocks of composite numbers.
Like? Share it with your friends
Facebook
Twitter
LinkedIn