Understanding Improper Fractions: Definition and Conversion
Table of Contents
Introduction
Improper Fractions
The concept of improper fractions plays a significant role in representing quantities greater than one in a fractional form. Let’s delve into the definition and conversion of improper fractions to gain a comprehensive understanding of their significance in mathematical contexts.
Analogy of Definition
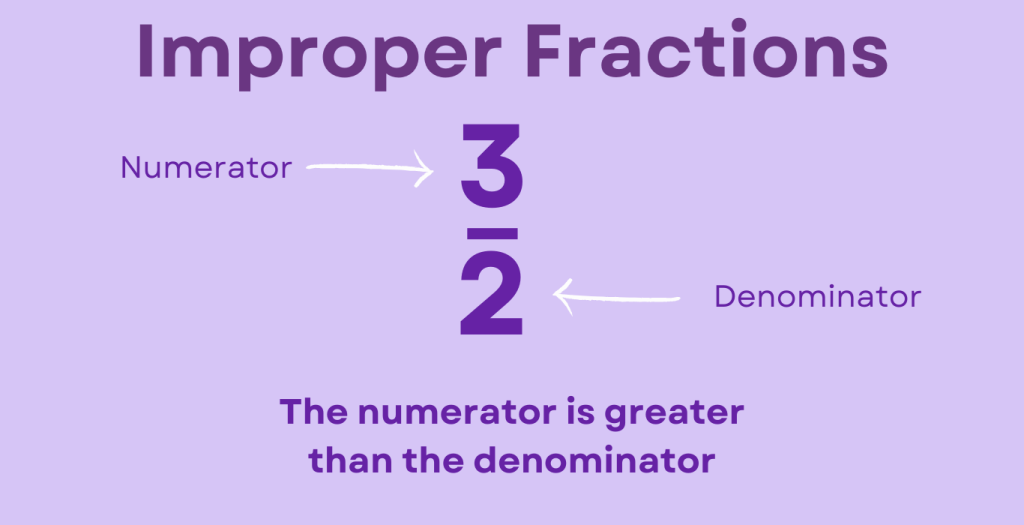
What are Improper Fractions?
An improper fraction is a type of fraction where the numerator is equal to or greater than the denominator, signifying a quantity greater than one. It is represented in the form of a fraction, showcasing the relationship between the numerator and denominator.
Method
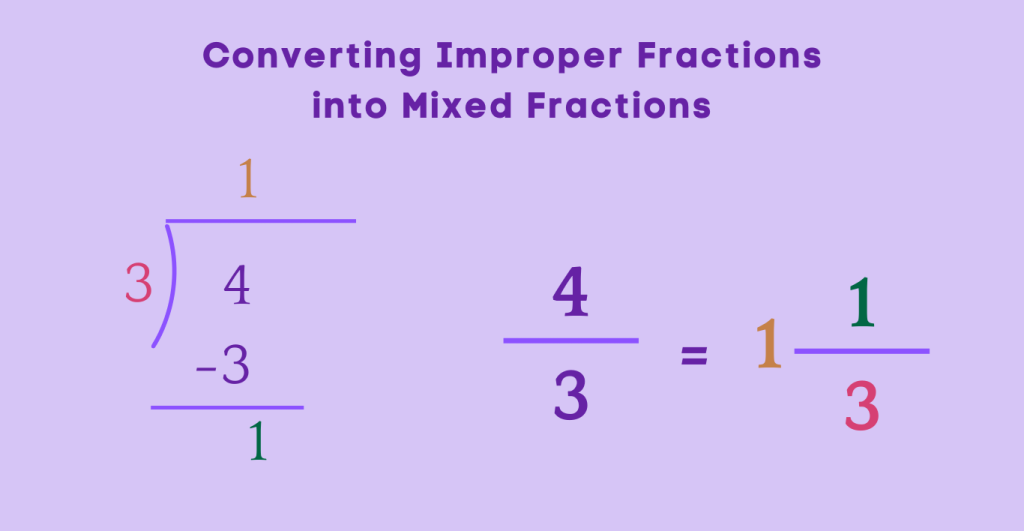
Converting Improper Fractions to Mixed Numbers
The process of converting an improper fraction to a mixed number involves dividing the numerator by the denominator and expressing the quotient as the whole number, with the remainder becoming the new numerator.
Examples
Example 1: Is \frac{5}{2} an improper fraction?
Answer:Â Since 7, the numerator is greater than the denominator, 3, it is an improper fraction.
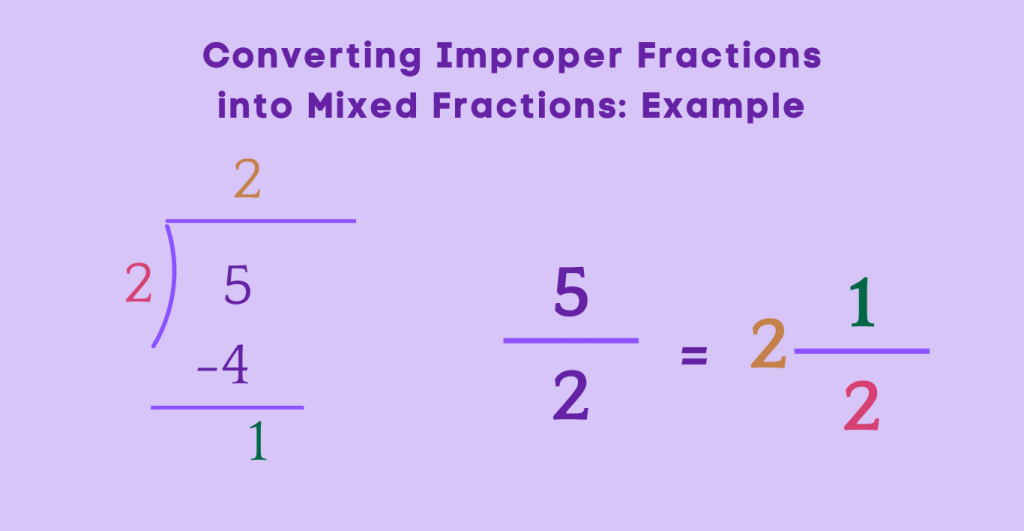
Example 2:Â Convert \frac{5}{2} into mixed fraction.
Quiz
Tips and Tricks
1. Identifying Improper Fractions
Tip: An improper fraction has a numerator (top number) that is greater than or equal to the denominator (bottom number).
2. Converting Mixed Numbers to Improper Fractions
Tip: Multiply the whole number by the denominator and add the numerator. This sum becomes the new numerator, while the denominator remains the same. For example to convert 3\frac{2}{5} into improper fraction, we will multiplyu 5 and 3 and add it with 2, 5 × 3 + 2 gives us 17, which will be the new numerator, so the improper fraction will be \frac{17}{5}.
3. Simplifying Improper Fractions
Tip: After performing operations, always check if the fraction can be simplified.
4. Visualizing Improper Fractions
Tip: Sometimes it helps to visualize the fraction using objects like pie charts or bars to understand why the numerator is larger than the denominator.
Real life application
Story: The Baking Adventure of Lily and Max
Lily and Max, two aspiring bakers, embarked on a baking adventure filled with challenges that required the application of improper fractions to achieve baking perfection.
Challenge 1: The Recipe Dilemma
Lily and Max encountered a recipe that called for 7/2 cups of sugar. Understanding the significance of improper fractions in recipes, they accurately measured 3 1/2 cups of sugar, ensuring the perfect sweetness in their baked goods.
Challenge 2: The Baking Proportions
In another baking endeavor, Lily and Max needed to adjust the recipe that required 9/4 cups of flour. By utilizing their knowledge of improper fractions, they measured 2 1/4 cups of flour, maintaining the ideal texture and consistency in their baked creations.
Challenge 3: The Financial Investment
In their final challenge, Lily and Max calculated the total cost of 11/3 units of a baking ingredient priced at $6 each. Applying their understanding of improper fractions in financial calculations, they estimated the cost to be $22, ensuring efficient budgeting and resource allocation for their baking venture.
FAQ's
An improper fraction is a fraction where the numerator is greater than or equal to the denominator. In other words, it represents a quantity greater than one.
To convert an improper fraction to a mixed number, divide the numerator by the denominator. The quotient becomes the whole number, and the remainder becomes the new numerator while the denominator remains the same.
Understanding improper fractions is crucial as it allows for the representation of quantities greater than one in a fractional form, enabling accurate mathematical calculations and problem-solving.
Yes, improper fractions can be simplified by finding their greatest common factor (GCF) and dividing both the numerator and denominator by it.
Improper fractions find practical applications in various real-life situations such as cooking recipes, measurements, and financial calculations.
Like? Share it with your friends
Facebook
Twitter
LinkedIn