Associative Property – Definition, Examples
Table of Contents
Introduction
Associative Property
In the realm of mathematics, the concept of the associative property plays a crucial role in understanding the behavior of operations such as addition, multiplication, and subtraction. This article delves into the intricacies of the associative property and its significance in mathematical operations.
Analogy of Definition
What is Associative Property?
The associative property is one of the fundamental number properties in mathematics that governs the grouping of numbers when performing operations. It states that the way in which numbers are grouped does not affect the result of the operation. In simpler terms, it means that the grouping of numbers can be changed without altering the outcome of the operation.
Method
Associative Property of Addition
The associative property of addition states that when adding three or more numbers, the grouping of the numbers does not affect the sum. In other words, the sum remains the same regardless of how the numbers are grouped.
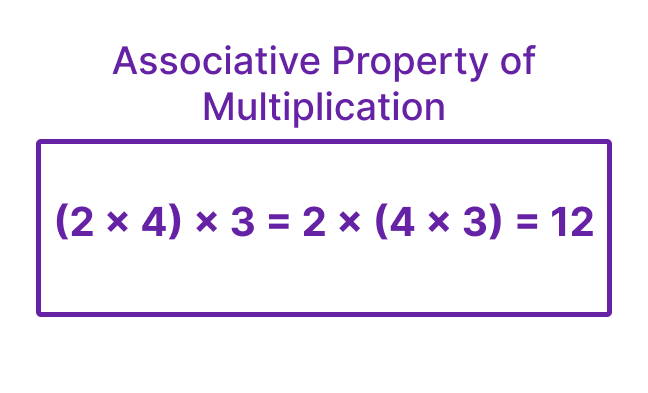
Associative Property of Multiplication
Similar to addition, the associative property of multiplication asserts that the grouping of numbers does not impact the product when multiplying three or more numbers. The product remains constant irrespective of the grouping of the numbers.
Associative Property of Subtraction
Let us check whether the associative property applied for subtraction or not with an example.
Let’s take, 6 – 7 – 5,
Using Associative Property, (6 – 7) – 5 should be equal to 6 – (7 – 5),
However, (6 – 7) – 5 = – 1 – 5 = – 6
and, 6 – (7 – 5) = 6 – 2 = 4
Hence, changing the grouping of the number gives us differeny results, so the associative property doesn’t apply.
Examples
Example 1: Associative Property of Addition
Consider the expression (2 + 3) + 4. According to the associative property of addition, the grouping of the numbers can be changed without altering the sum. Therefore, (2 + 3) + 4 = 2 + (3 + 4) = 9.
Example 2: Associative Property of Multiplication
For the expression (5 × 2) × 3, the associative property of multiplication allows us to regroup the numbers as 5 × (2 × 3), resulting in the same product of 30.
Quiz
Tips and Tricks
1.Understanding the Associative Property
Tip: The associative property states that the way in which numbers are grouped in an addition or multiplication problem does not change the result. In other words, you can change the grouping of the numbers without changing the outcome.
2.Visualizing Grouping
Tip: Imagine grouping numbers differently within an addition or multiplication problem. The result will remain the same regardless of how the numbers are grouped together.
3.Applying to Addition
Tip: For addition, the associative property can be illustrated by rearranging the order of the numbers being added together. For example, (2 + 3) + 4 is equal to 2 + (3 + 4), demonstrating that the grouping doesn’t affect the sum.
4.Applying to Multiplication
Tip: Similarly, the associative property applies to multiplication. You can rearrange the order of multiplication without changing the product. For instance, (2 × 3) × 4 is equal to 2 × (3 × 4), showing that the grouping doesn’t affect the product.
5.Using Parentheses
Tip: Parentheses are often used to illustrate grouping in math problems. Understanding the associative property allows you to simplify expressions by rearranging the parentheses while maintaining the same result.
Real life application
Story: “The Math Challenge of Maya and Ethan”
Maya and Ethan, two enthusiastic students, encountered real-life scenarios where the associative property played a pivotal role in solving mathematical challenges.
Challenge 1: The Group Project
Maya and Ethan were assigned a group project that involved adding multiple sets of numbers. By applying the associative property of addition, they were able to rearrange the numbers and streamline their calculations, ensuring accuracy and efficiency in their project.
Challenge 2: The Budget Analysis
In a budget analysis task, Maya and Ethan encountered various multiplication operations. By leveraging the associative property of multiplication, they were able to regroup the numbers and simplify the calculations, leading to a comprehensive analysis of the budget.
Challenge 3: The Inventory Management
When dealing with subtraction operations in inventory management, Maya and Ethan utilized the associative property of subtraction to ensure consistency in their calculations. By appropriately grouping the numbers, they maintained accuracy in tracking inventory levels.
FAQ's
The associative property ensures that the grouping of numbers does not affect the result of addition, multiplication, and, when appropriately used, subtraction. It provides a framework for rearranging numbers to simplify calculations and maintain consistency in outcomes.
While the associative property is commonly associated with addition and multiplication, it can also be applied to subtraction by using parentheses to group the numbers. This allows for the application of the associative property to ensure consistent results in subtraction operations.
Understanding the associative property enables individuals to streamline mathematical operations, simplify complex expressions, and maintain accuracy in calculations. It provides a foundational understanding of how numbers can be grouped to achieve consistent results.
The associative property has practical applications in various real-life situations, such as budget analysis, inventory management, and group projects. By leveraging the associative property, individuals can simplify calculations and ensure accuracy in mathematical operations.
The application of the associative property involves recognizing the grouping of numbers and understanding how the property can be utilized to simplify mathematical expressions. Whether it’s addition, multiplication, or subtraction, the associative property provides a framework for rearranging numbers to streamline calculations.
Like? Share it with your friends
Facebook
Twitter
LinkedIn