What is Distributive Property?
Table of Contents
Introduction
Distributive Property
In the realm of mathematics, the distributive property plays a crucial role in simplifying expressions and solving equations. It is a fundamental concept that is widely used in various mathematical operations. Let’s delve into the world of the distributive property and understand its significance in mathematical calculations.
Analogy of Definition
What is Distributive Property?
The distributive property of multiplication over addition states that for any three numbers a, b, and c, the product of a and the sum of b and c is equal to the sum of the products of a and b, and a and c. In mathematical terms, it can be represented as: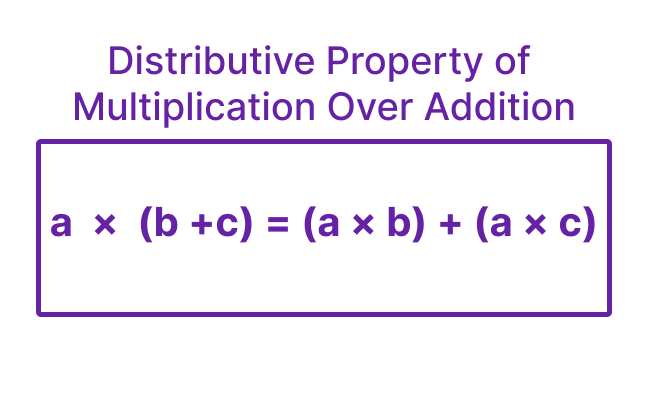
Similarly, the distributive property of multiplication over subtraction states that for any three numbers a, b, and c, the product of a and the difference of b and c is equal to the difference of the products of a and b, and a and c. In mathematical terms, it can be represented as:
Method
Verification of the Distributive Property
To verify the distributive property, one can choose any set of numbers and perform the calculations using both the original expression and the expression obtained by applying the distributive property. By comparing the results, one can confirm the validity of the distributive property.
For instance, let’s take an example of 5 × (3 + 2)
Solving without applying distributive property:
5 × (3 + 2) = 5 × 5 = 25
Solving with applying distributive property:
5 × (3 + 2) = (5 × 3 ) + (5 × 2) = 15 + 10 = 25
Hence, we can verify the distributive property.
How to Use the Distributive Property
When faced with mathematical expressions involving multiplication and addition or subtraction, the distributive property can be applied to simplify the expressions and make calculations more manageable. By distributing the multiplication across the terms within the parentheses, complex expressions can be broken down into simpler forms.
Examples
Example 1: Distributive Property of Multiplication over Addition
Given: 3 × (4 + 5)
Using the distributive property: 3 × 4 + 3 × 5
Result: 12 + 15 = 27
Example 2: Distributive Property of Multiplication over Subtraction
Given: 3 × (7 – 2)
Using the distributive property: 3 × 7 – 3 × 2
Result: 21 – 6 = 15
Quiz
Tips and Tricks
1. Using the distributive property of multiplication over addition.
Tip: To apply the distributive property, distribute the multiplication across the terms within the parentheses and then perform the addition.
Calculation: 8 × (12 + 3) = 8 × 12 + 3 × 8
Result: 96 + 24 = 120
2.Using the distributive property of multiplication over subtraction.
Tip: When using the distributive property over subtraction, distribute the multiplication across the terms within the parentheses and then perform the subtraction.
Calculation: 10 × (5 – 2) = 5 × 10 – 2 × 10
Result: 50 – 20 = 30
3. Use Parentheses
Tip: Parentheses are commonly used to represent the terms being multiplied together. Recognize that the distributive property allows you to remove the parentheses by distributing the multiplication to each term inside.
Real life application
Story: “The Distributive Property Adventures of Maya and Ethan”
Maya and Ethan, two young math enthusiasts, embarked on a series of real-life challenges that required them to apply the distributive property to solve practical problems.
Challenge 1: The Lemonade Stand
Maya and Ethan decided to set up a lemonade stand to raise funds for a local charity. They needed to calculate the total cost of purchasing lemons and sugar. By using the distributive property of multiplication over addition, they determined the total cost of 5 bags of lemons at $3 per bag and 3 bags of sugar at $2 per bag, resulting in a total cost of $21.
Challenge 2: The Art Supplies Purchase
As part of a school project, Maya and Ethan needed to buy art supplies. They had to calculate the total cost of 8 packs of colored pencils at $5 per pack and 4 packs of sketchbooks at $7 per pack. By applying the distributive property of multiplication over addition, they found the total cost to be $76.
Challenge 3: The Charity Bake Sale
Maya and Ethan volunteered to organize a charity bake sale. They had to calculate the total revenue from selling 6 boxes of cookies at $8 per box and 3 boxes of brownies at $6 per box. Using the distributive property of multiplication over addition, they determined the total revenue to be $66.
FAQ's
The distributive property is applied in real-life scenarios to calculate the total cost, revenue, or refunds when dealing with multiple items or products. It simplifies the process of performing calculations involving multiplication and addition or subtraction, making it easier to manage financial transactions and resource allocation.
The distributive property provides a systematic approach to simplify complex expressions and equations, making mathematical calculations more efficient. It allows for the transformation of mathematical operations involving multiplication and addition or subtraction, leading to accurate results and streamlined problem-solving.
Yes, the distributive property is a fundamental concept in algebra and is used to simplify algebraic expressions by distributing the multiplication across the terms within the parentheses. This simplification aids in solving equations and manipulating algebraic formulas.
Yes, the distributive property can be verified by choosing arbitrary numbers and performing the calculations using both the original expression and the expression obtained by applying the distributive property. By comparing the results, one can confirm the validity of the distributive property.
The distributive property of multiplication over subtraction involves distributing the multiplication across the terms within the parentheses and then performing subtraction, while the distributive property of multiplication over addition involves distributing the multiplication and then performing addition. Both properties follow the same fundamental concept but differ in the operation performed after distribution.
Like? Share it with your friends
Facebook
Twitter
LinkedIn