How to add fractions? – Step-by-Step Guide

Approaches to Adding Fractions

There are several methods with which we can add fractions, depending on the type of fractions being added. The methods include adding fractions with like denominators, adding fractions with unlike denominators, adding fractions with whole numbers, and adding mixed fractions. Each scenario requires a specific approach to ensure accurate and efficient addition.

Step-by-Step Guide

How to Add Fractions with Same Denominators?

When adding fractions with like denominators, simply add the numerators together while keeping the denominator unchanged.

For example, when adding \frac{1}{4} and  \frac{3}{24} ,we get, 

\frac{1}{4} + \frac{3}{4} = \frac{4}{4} = 1  

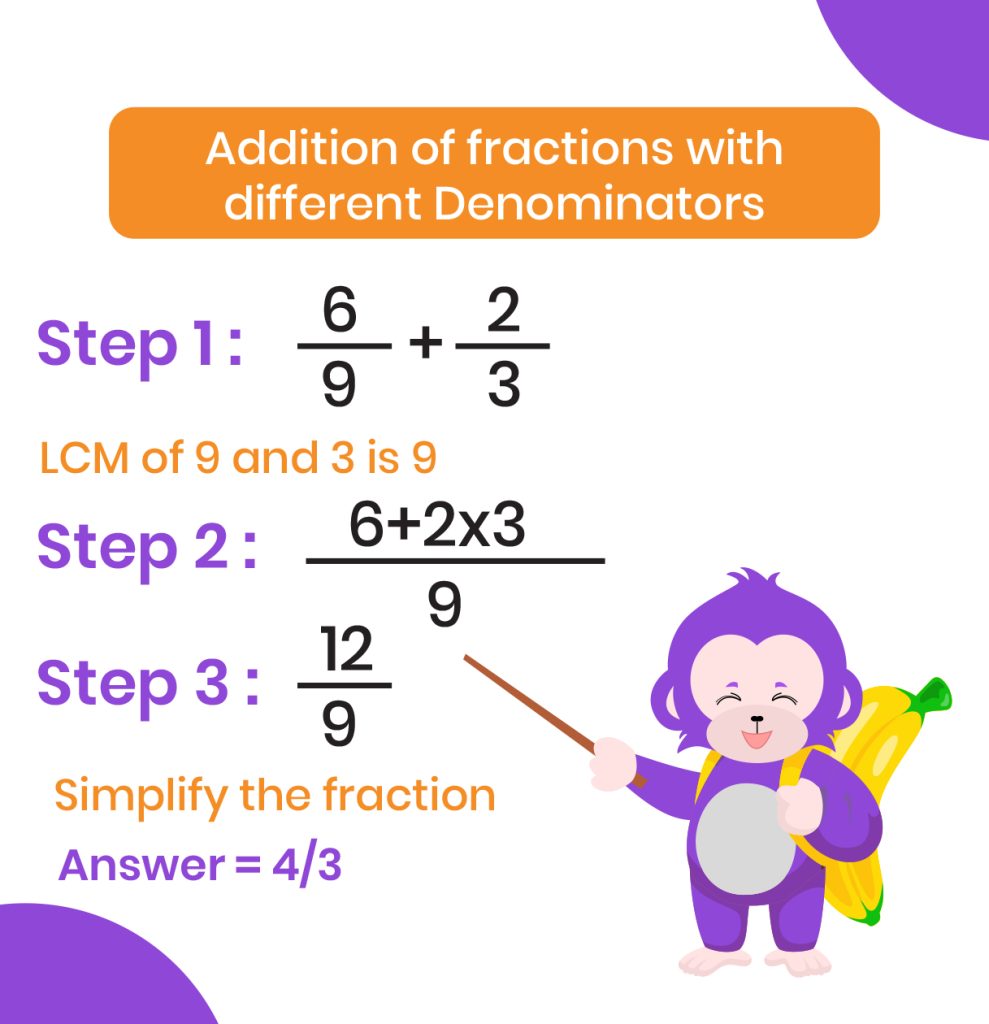
How to Add Fractions with Different Denominators? 

When adding unlike fractions or fractions with different denominators, we first, make the denominators equivalent by finding the least common multiple (LCM) of the denominators. Then, we convert each fraction to an equivalent fraction with the common denominator and proceed to add the numerators.

Lets’s add \frac{6}{9} and \frac{2}{3}

\frac{6}{9} + \frac{2}{3}

We can see that the fractions have different denominators, meaning they are unlike fractions.

Step 1: To solve the problem, we will first find the LCM of the denominators. The LCM of 3 and 9 = 9

Step 2: To convert the fractions into like fractions, we will have to multiply \frac{2}{3} with \frac{3}{3}. \frac{2}{3} × \frac{3}{3} = \frac{6}{3}

Step 3: Now that both the fractions are like, we will add them. \frac{6}{9} + \frac{6}{9} = \frac{12}{9}

Step 4: As we can simply \frac{12}{9}, our final answer will be \frac{4}{3}

Adding Fractions with Whole Numbers

When adding fractions with whole numbers, convert the whole numbers to fractions with the denominator of 1. Then, proceed to make the denominators same and add the fraction. 

For example: Let’s add \frac{4}{3} with 3

Step 1: We will first write 3 in a fraction form with 1 as the denominator as \frac{3}{1}

Step 2: We will then multiply \frac{3}{1} with 3, to get the same denominator. We will get \frac{3}{1}  × \frac{3}{3} = \frac{9}{3}

Step 3: Now, adding \frac{4}{3} with \frac{9}{3} , we get \frac{4}{3} + \frac{9}{3}  = \frac{13}{3}

Adding Mixed Fractions

To add mixed fractions, first, convert the mixed fractions to improper fractions. Then, proceed to add the fractions as usual, ensuring that the final sum is simplified to its simplest form if necessary.

Lets add, 1\frac{1}{4} + 2\frac{1}{3}

Step 1: First, we will convert the mixed fraction into improper fraction as \frac{4*1+1}{4} +  \frac{3*2+1}{4} = \frac{5}{4} +\frac{7}{3} 

Step 2: We will then add the converted fraction \frac{5*3}{12} + \frac{7*4}{12} = \frac{15}{12}+\frac{28}{12} = \frac{43}{12}

Learn more about fractions on our site, visit ChimpVine.