Understanding Perfect Squares
Table of Contents
Introduction
Understanding Perfect Squares
Exploring the concept of perfect squares unveils the fascinating world of numbers and their intriguing properties. Let’s delve into the realm of perfect squares and unravel their significance in the realm of mathematics.
Analogy of Definition
What are Perfect Squares?
A perfect square is a number that is the result of multiplying an integer (a whole number) by itself. In other words, if you take a whole number and multiply it by itself, you get a perfect square.
For example:
- 1 × 1 = 1
- 2 × 2 = 4
- 3 × 3 = 9
- 4 × 4 = 16
- 5 × 5 = 25
So, 1, 4, 9, 16, and 25 are all perfect squares.
Method
Identifying Perfect Squares
To identify a perfect square, one can take the square root of a number and check if the result is a whole number. If the square root is an integer, then the original number is a perfect square.
- Multiplication Method
- Multiply a whole number by itself. If the result matches the number you’re checking, then it is a perfect square.
- Example: To check if 36 is a perfect square, see if there is a whole number that, when multiplied by itself, equals 36. Since 6×6=36, 36 is a perfect square.
- Square Root Method
- Find the square root of the number. If the square root is a whole number, then the original number is a perfect square.
- Example: The square root of 49 is 7, which is a whole number, so 49 is a perfect square.
- Units Digit Rule:
- Look at the last digit (units digit) of the number. A perfect square’s units digit must be 0, 1, 4, 5, 6, or 9.
- Example: The number 64 ends in 4, which is one of the possible units digits for perfect squares. (Further checking shows 8×8=64, confirming it is a perfect square.)
- Sum of Consecutive Odd Numbers:
- A perfect square can be expressed as the sum of consecutive odd numbers starting from 1.
- Example: 1 + 3 + 5 + 7 = 16, so 16 is a perfect square.
Examples
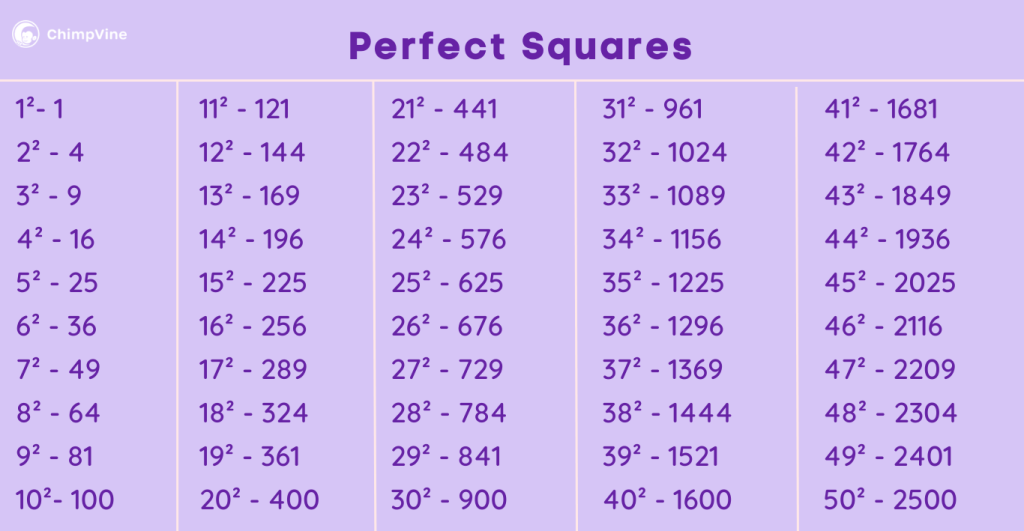
Quiz
Tips and Tricks
1. Identifying Perfect Squares
Tip: To identify a perfect square, take the square root of the number and check if the result is a whole number.
2. Units Digit Check
Tip:A perfect square always ends in 0, 1, 4, 5, 6, or 9. Example: If a number ends in 2, 3, 7, or 8, it cannot be a perfect square.
3. Square Root Shortcut
Tip: To quickly find if a number is a perfect square, estimate its square root. If the square root is a whole number, the number is a perfect square. Example: For 81, estimate the square root (between 8 and 9). Since 9 × 9 = , it is a perfect square.
4. Sum of Odd Numbers
Tip: Any perfect square is the sum of consecutive odd numbers. Example: 1+3+5+7+9=25, so 25 is a perfect square.
5. Difference of Squares
Tip:The difference between consecutive perfect squares is always an odd number. Example: 5² – 4² = 25 – 16 = 9 (an odd number).
Real life application
Story: The Perfect Square Adventure of Alex and Sophia
Alex and Sophia embarked on an adventure filled with challenges that required the application of perfect squares to overcome obstacles and achieve success.
Challenge 1: The Garden Design Quest
Alex and Sophia were tasked with designing a garden with a perfect square shape. By applying their knowledge of perfect squares, they calculated the area of the garden and successfully completed the quest.
Challenge 2: The Puzzle Challenge
Continuing their adventure, Alex and Sophia encountered a series of puzzles that involved identifying perfect squares. By utilizing their understanding of perfect squares, they swiftly solved the puzzles and progressed further in their journey.
Challenge 3: The Real-World Applications
In their final challenge, Alex and Sophia encountered real-life scenarios where the concept of perfect squares was instrumental. From calculating areas of squares to determining distances in coordinate geometry, they applied their knowledge of perfect squares to overcome challenges and emerge victorious.
FAQ's
A perfect square is a number that can be expressed as the product of an integer multiplied by itself. In other words, it is the square of a whole number.
Perfect squares are essential in various mathematical concepts such as geometry, algebra, and number theory. They are fundamental in understanding the properties of numbers and their relationships.
To identify a perfect square, you can take the square root of a number. If the result is a whole number, then the original number is a perfect square.
Perfect squares have unique properties, including being non-negative, having an odd number of factors, and being the product of two identical factors.
Perfect squares find practical applications in various real-life situations such as calculating areas of squares, determining distances in coordinate geometry, and solving problems in physics and engineering.
Like? Share it with your friends
Facebook
Twitter
LinkedIn