What is a Polygon? – Shapes, Angles and Example
Table of Contents
Introduction
Polygons
In the realm of geometry, the term “polygon” is a fundamental concept that encompasses a wide range of shapes and structures. Understanding the various types of polygons, their sides, and angles is essential in exploring the intricacies of geometric figures and their properties.
Analogy of Definition
What is a Polygon?
A polygon is a two-dimensional closed figure formed by three or more line segments called sides. These sides intersect only at their endpoints, creating a series of angles within the figure. Polygons can vary in complexity, and their properties are determined by the number of sides, the types of angles, and the arrangement of vertices.
Method
Types of Polygons
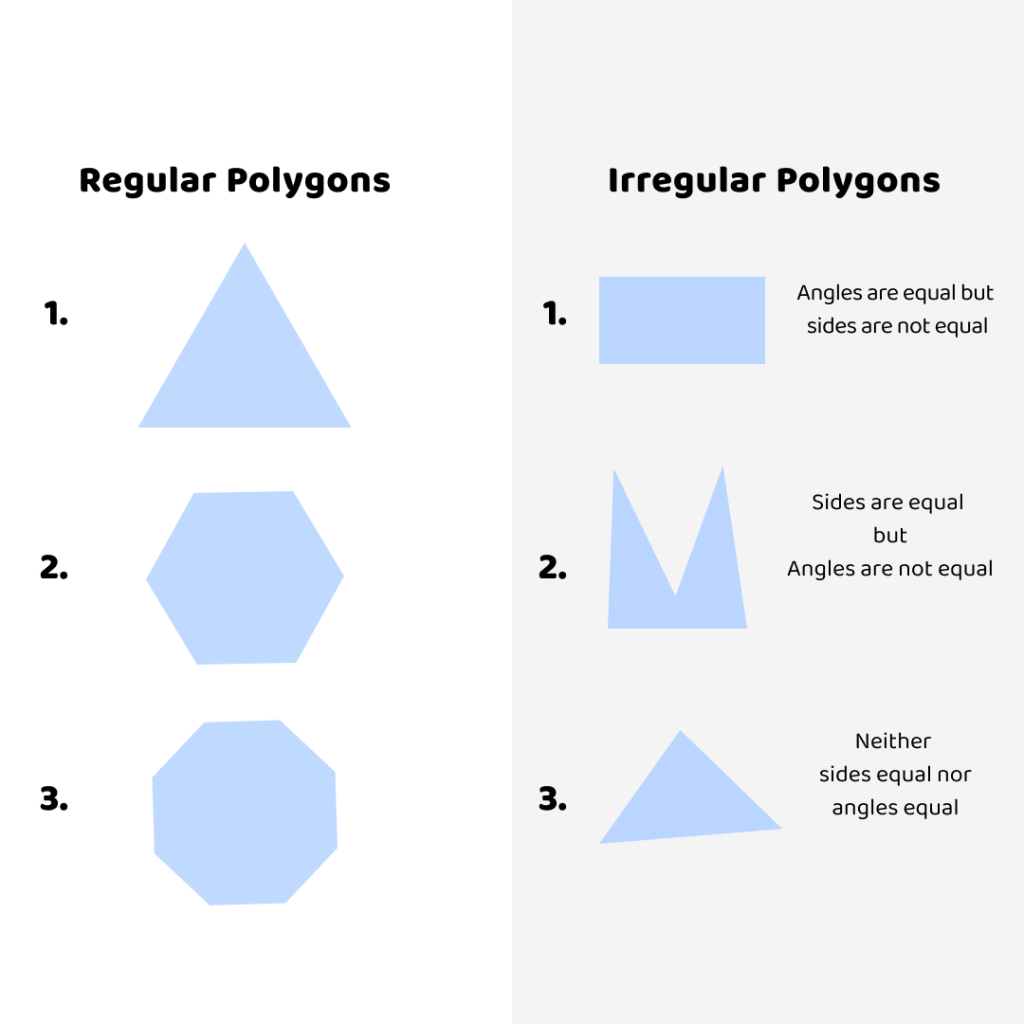
1. Regular and Irregular Polygons
Regular Polygon: A polygon with all sides of equal length and all angles of equal measure.
Irregular Polygon: A polygon with sides and/or angles of different lengths and measures.
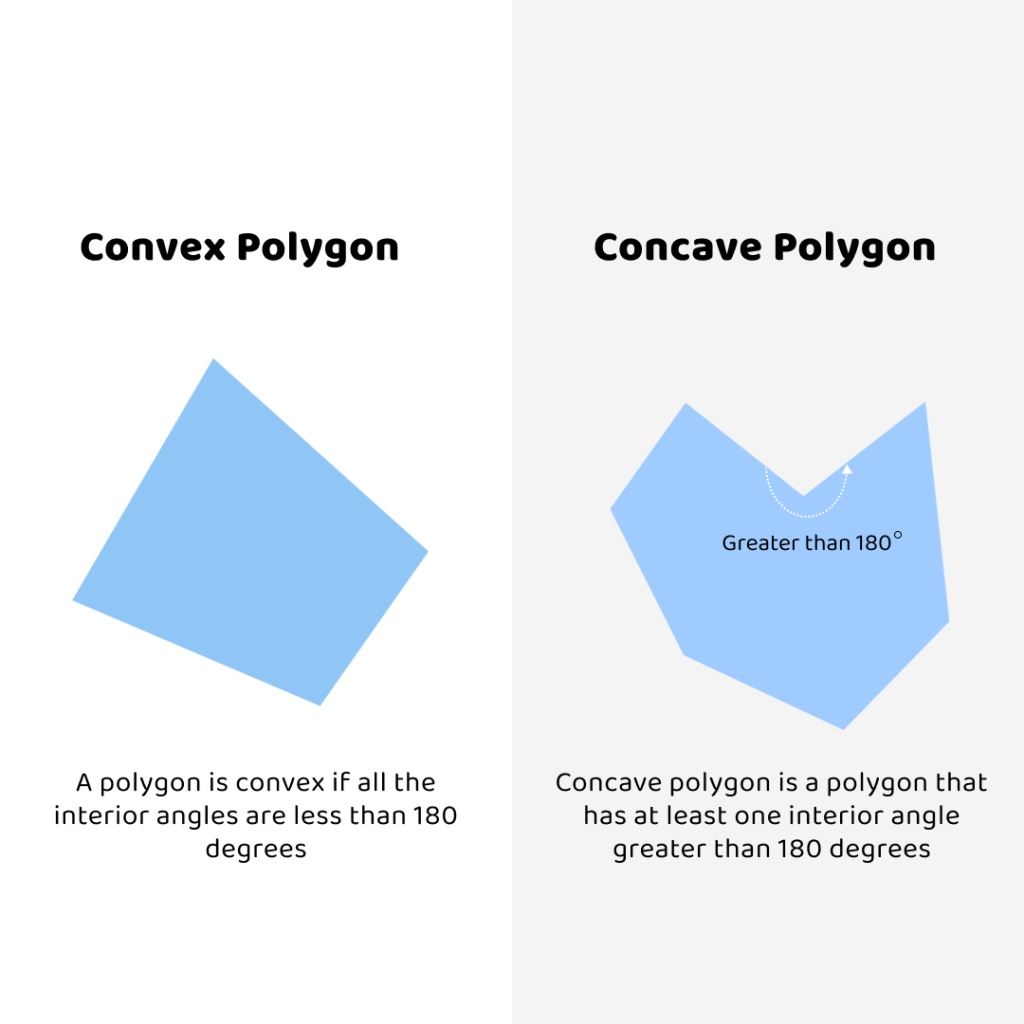
2. Convex and Concave Polygons
Convex Polygon: A polygon in which all interior angles are less than 180 degrees, and all line segments connecting any two points within the polygon lie entirely inside the figure.
Concave Polygon: A polygon with at least one interior angle greater than 180 degrees, causing some line segments to extend outside the figure.
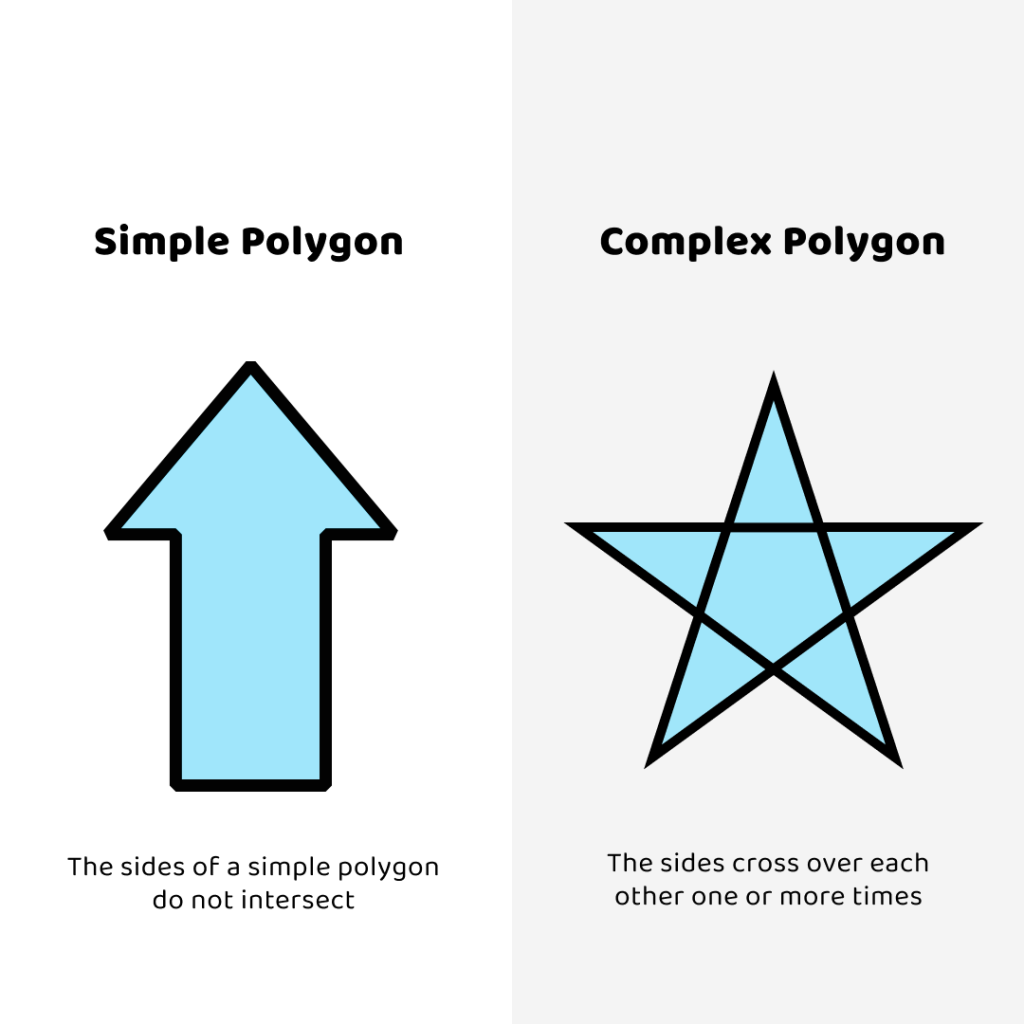
3. Simple and Complex Polygons
Simple Polygon: A polygon with non-intersecting sides and angles, forming a single, continuous boundary.
Complex Polygon: A polygon with intersecting sides and angles, resulting in multiple boundaries and internal regions.
Types of Polygons Based on Sides
1. Triangle
A polygon with three sides and three angles.
2. Quadrilateral
A polygon with four sides and four angles.
3. Pentagon
A polygon with five sides and five angles.
4. Hexagon
A polygon with six sides and six angles.
5. Heptagon
A polygon with seven sides and seven angles.
Angles of Polygons
The sum of the interior angles of a polygon can be calculated using the formula (n-2) * 180 degrees, where n represents the number of sides in the polygon. Each interior angle of a regular polygon can be found by dividing the sum of the interior angles by the number of sides.
In a polygon, the sum of exterior angle and interior angle should be equal to 180°. Hence, to find out the exterior angle of triangle, the formula will be as follows.
To learn more about polygon with interactive activities, visit our site ChimpVine.
Examples
Finding out angles of Polygons
Example 1: If a polygon has 4 sides, what will be the sum of its interior angles?
Sum of interior angles of polygon = (n – 2) * 180° = (4 – 2) * 180° = 2 * 180° = 360°
Example 2: If a polygon has 4 sides, and one of its interior angles is 90°, what will be the exterior angle of its vertices?
Exterior Angle = 180° – interior angle = 180° – 90° = 90°
Quiz
Tips and Tricks
1. Identifyindg Regular Polygons
Tip: A regular polygon has all sides of equal length and all angles of equal measure. Examples include equilateral triangles, squares, and regular pentagons.
2. Understanding Interior Angles
Tip: The sum of the interior angles of a polygon can be calculated using the formula (n-2) * 180 degrees, where n represents the number of sides in the polygon.
3. Classifying Convex and Concave Polygons
Tip: A convex polygon has all interior angles less than 180 degrees, while a concave polygon has at least one interior angle greater than 180 degrees.
4. Recognizing Simple and Complex Polygons
Tip: A simple polygon has non-intersecting sides and angles, forming a single, continuous boundary. A complex polygon has intersecting sides and angles, resulting in multiple boundaries and internal regions.
5. Identifying Irregular Polygons
Tip: An irregular polygon has sides and/or angles of different lengths and measures, making it distinct from regular polygons with uniform properties.
Real life application
Story: “The Polygon Adventures of Maya and Ethan”
Maya and Ethan, two young explorers, embarked on a series of adventures that required them to apply their knowledge of polygons in real-life scenarios.
Adventure 1: The Architect’s Blueprint
Maya and Ethan visited an architect’s office, where they learned about designing buildings with various polygonal shapes. They observed that regular polygons, such as squares and equilateral triangles, were commonly used in architectural blueprints to create symmetrical and structurally sound structures.
Adventure 2: The Garden Design Challenge
In a botanical garden, Maya and Ethan encountered a garden design challenge that involved arranging flower beds in the shape of different polygons. They used their understanding of polygon sides and angles to create visually appealing patterns, incorporating triangles, pentagons, and hexagons into the garden layout.
Adventure 3: The Geometric Art Exhibition
At an art exhibition, Maya and Ethan explored geometric art installations featuring complex polygons. They appreciated the intricate designs created by intersecting sides and angles, showcasing the beauty of complex polygonal shapes in artistic expressions.
FAQ's
Polygons can be classified based on the number of sides they possess, ranging from triangles (3 sides) to heptagons (7 sides) and beyond. Each type of polygon exhibits unique properties and geometric characteristics.
The number of sides in a polygon determines the sum of its interior angles. The relationship between the sides and angles of a polygon is governed by mathematical formulas and principles, allowing for the calculation and analysis of polygonal properties.
A regular polygon has all sides of equal length and all angles of equal measure, resulting in a symmetrical and uniform shape. In contrast, an irregular polygon exhibits variations in side lengths and angle measures, leading to asymmetrical and non-uniform configurations.
The distinction between convex and concave polygons is relevant in fields such as architecture, urban planning, and industrial design. Convex polygons are preferred for their stability and uniformity, while concave polygons may present challenges in structural integrity and spatial organization.
Polygons play a crucial role in diverse real-life applications, including architectural design, urban planning, art and aesthetics, landscaping, and geometric modeling. Their properties and configurations influence the visual, structural, and functional aspects of various objects and environments.
Like? Share it with your friends
Facebook
Twitter
LinkedIn