Understanding Irrational Numbers: Definition and Properties
Table of Contents
Introduction
Irrational Numbers
In the realm of mathematics, the concept of irrational numbers holds significant importance. Let’s delve into the definition and properties of irrational numbers, exploring their unique characteristics and real-world applications.
Analogy of Definition
What are Irrational Numbers?
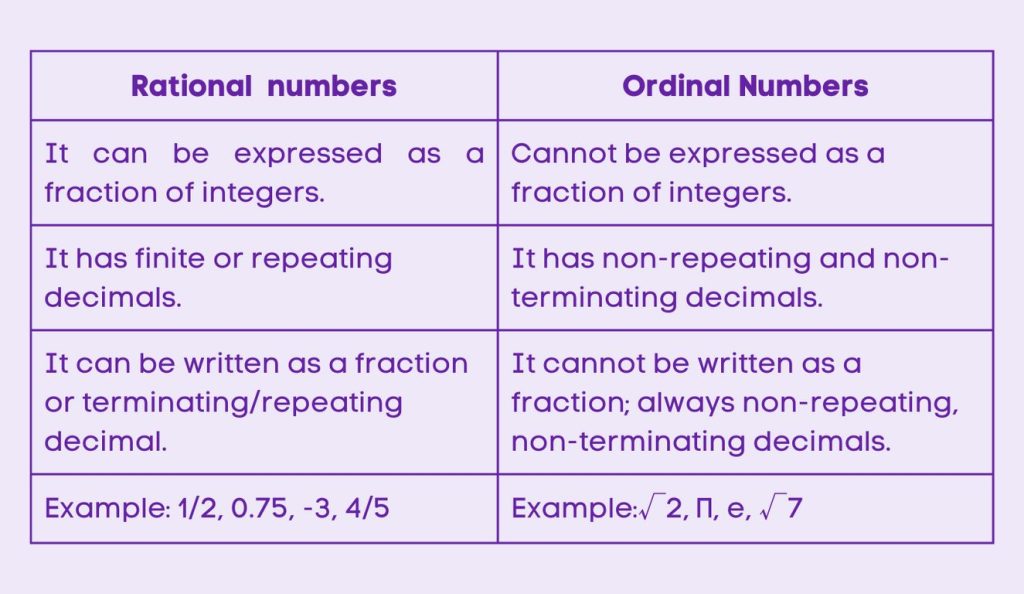
Irrational numbers are real numbers that cannot be expressed as a fraction of two integers. Unlike rational numbers, irrational numbers have non-repeating, non-terminating decimal representations, making them distinct from their rational counterparts.
Method
Properties of Irrational Numbers
Irrational numbers possess distinct properties, including non-repeating and non-terminating decimal expansions, the inability to be expressed as a simple fraction, and the presence of an infinite number of non-repeating digits after the decimal point.
1. Non-Terminating and Non-Repeating Decimals: Irrational numbers cannot be expressed as terminating decimals (decimals that end) or repeating decimals (decimals with a repeating pattern).
2. Infinite Decimal Expansion: The decimal expansion of an irrational number goes on forever without repeating any pattern.
3. Cannot be Written as Fractions: Irrational numbers cannot be expressed as a ratio of two integers. In other words, they cannot be written in the form a/b, where a and b are integers and b is not zero.
4. Examples: Common examples of irrational numbers include the square root of non-perfect squares (e.g., √2, √3, √5), transcendental numbers (e.g., π, e), and many square roots of prime numbers.
5. Density in the Real Number Line: Irrational numbers are densely populated on the real number line, meaning between any two rational numbers, there exists an irrational number.
6. Not Closed Under Addition or Multiplication: The sum or product of two irrational numbers is not necessarily irrational. For example, √2 + √2 = 2√2 (which is irrational), but √2 * √2 = 2 (which is rational).
Rational and Irrational Numbers
Examples
Example 1: The square root of 2 (√2) is an irrational number, as it exhibits a non-repeating, non-terminating decimal expansion.
Example 2: The value of pi (π) is another example of an irrational number, with its decimal representation extending infinitely without repetition.
Example 3: The golden ratio (φ) is an irrational number that appears in various natural and artistic contexts, characterized by its non-repeating, non-terminating decimal expansion.
Summary:
The examples of irrational numbers, such as the square root of 2, the value of pi, and the golden ratio, exemplify the unique properties of irrational numbers, including non-repeating, non-terminating decimal expansions and the inability to be expressed as a simple fraction. These examples illustrate the distinct nature of irrational numbers compared to rational numbers, shedding light on their significance in mathematical and real-world contexts.
The examples of irrational numbers, such as the square root of 2, the value of pi, and the golden ratio, exemplify the unique properties of irrational numbers, including non-repeating, non-terminating decimal expansions and the inability to be expressed as a simple fraction. These examples illustrate the distinct nature of irrational numbers compared to rational numbers, shedding light on their significance in mathematical and real-world contexts.
Quiz
Tips and Tricks
1. Identifying Irrational Numbers
Tip: If a number cannot be expressed as a fraction of two integers and has a non-repeating, non-terminating decimal expansion, it is irrational.
2. Real-World Applications
Tip: Irrational numbers, such as the value of pi and the golden ratio, are prevalent in geometric calculations and artistic designs.
3. Properties of Irrational Numbers:
Tip: Irrational numbers possess non-repeating and non-terminating decimal expansions, along with the inability to be expressed as a simple fraction.
Real life application
Story: The Architect’s Design Dilemma
An architect faced a design challenge that required the use of the golden ratio, an irrational number, to achieve aesthetic harmony and balance in a building’s proportions. By incorporating the golden ratio into the design, the architect created a visually appealing structure that captivated onlookers and exemplified the application of irrational numbers in architecture and design.
FAQ's
Irrational numbers are real numbers that cannot be expressed as a fraction of two integers. In contrast, rational numbers can be expressed as a fraction of two integers. The key distinction lies in the fact that irrational numbers have non-repeating, non-terminating decimal representations, while rational numbers have either terminating or repeating decimals.
Irrational numbers possess several unique properties, including the inability to be expressed as a simple fraction, non-repeating and non-terminating decimal expansions, and the presence of an infinite number of non-repeating digits after the decimal point. Additionally, the sum, difference, product, or quotient of an irrational number with a rational number is always irrational.
Yes, irrational numbers can be located on the number line between any two rational numbers. They are interspersed throughout the number line and are not confined to specific intervals or regions.
Irrational numbers find practical applications in various fields such as geometry, physics, and engineering. For instance, the value of pi (π) is an irrational number and is utilized in calculations involving circles and spheres. Additionally, the golden ratio, another irrational number, is prevalent in art, architecture, and design.
Examples of irrational numbers include the square root of non-perfect squares (e.g., √2, √3, √5), the value of pi (π), and the golden ratio (φ). These numbers exhibit non-repeating, non-terminating decimal expansions and cannot be expressed as a simple fraction.
Like? Share it with your friends
Facebook
Twitter
LinkedIn