How to Find Radius from Circumference – Conversion and Formula

Introduction

Circumference and Radius

When dealing with circles, understanding the formula to find out radius from circumference is essential. The circumference is the distance around the circle, while the radius is the distance from the center to any point on the circle. Let’s explore the conversion and formula for finding the radius from the circumference.

Analogy of Definition

Radius

The radius of a circle is the distance from the center of the circle to any point on its edge. It’s a straight line segment that connects the center of the circle to its perimeter. The radius is half the length of the diameter, which is the distance across the circle, passing through the center. The radius is a key measurement in understanding the size and properties of a circle.

Circumference

The circumference of a circle is the total distance around the edge of the circle. It’s essentially the perimeter of the circle. You can think of it as the length of the circle if you were to cut it and lay it out in a straight line. The circumference is directly related to the radius and diameter of the circle, and it can be calculated using the formula $C = 2 π r$ , where $C$ represents the circumference, is the radius, and $π$ (pi) is a mathematical constant approximately equal to 3.14159. Based on the same formula, we can find out radius from circumference.

Method

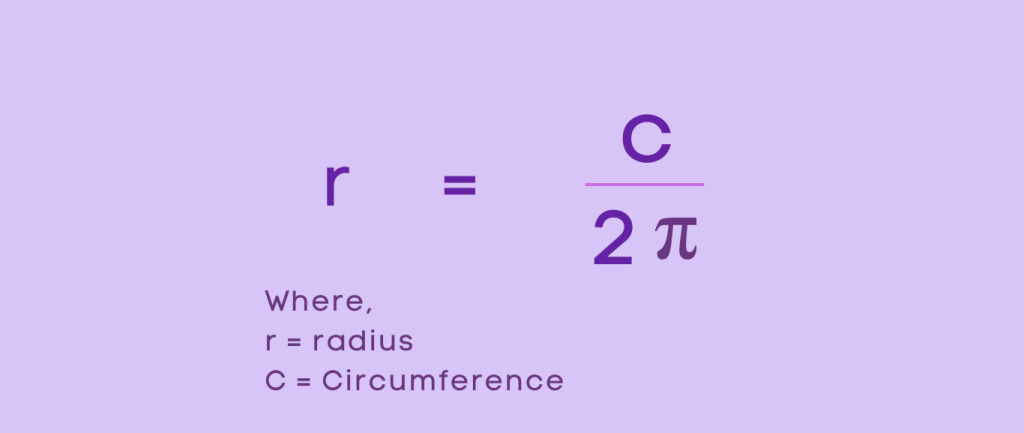
Radius from Circumference Formula

Finding out radius from circumference involves using the formula r = C / (2π), where r is the radius, C is the circumference, and π is the mathematical constant pi. This formula allows for the calculation of the radius based on the given circumference.

Examples

Radius from Circumference – Example 1:

Given Circumference: 12π units
Calculation: r = 12π / (2π) = 6 units
Result: The radius of the circle is 6 units.

Radius from Circumference – Example 2:

Given Circumference: 18π units
Calculation: r = 18π / (2π) = 9 units
Result: The radius of the circle is 9 units.

Radius from Circumference – Example 3:

Given Circumference: 20π units
Calculation: r = 20π / (2π) = 10 units
Result: The radius of the circle is 10 units.

Summary:
These examples illustrate the process of finding the radius from the given circumferences of circles. By applying the formula r = C / (2π), the radius can be calculated efficiently, allowing for the determination of the distance from the center to any point on the circle.

Quiz

Tips and Tricks

1. Understanding the Formula

Tip: To find the radius from circumference, use the formula r = C / (2π), where r is the radius, C is the circumference, and π is the mathematical constant pi.

2. Applying the Conversion

Tip: When given the circumference, utilize the formula r = C / (2π) to calculate the radius of the circle.

3. Practical Application

Tip: Understanding the relationship between circumference and radius is essential for various real-life applications involving circles.

Real life application

Scenario: Construction Project
In a construction project, finding out radius from circunference is crucial for determining the dimensions of circular structures such as columns and arches. By calculating the radius from the given circumference, accurate measurements can be obtained for construction purposes.

Scenario: Engineering Design
In engineering design, the finding out radius from circumferenceis utilized to create precise circular components for machinery and equipment. Understanding this relationship allows engineers to design and fabricate circular parts with the required dimensions.

Scenario: Art and Design
Artists and designers often use the conversion from circumference to radius to create circular patterns and designs. By calculating the radius from the given circumference, they can achieve symmetry and precision in their artistic creations.

FAQ's

What is the relationship between circumference and radius?

The circumference of a circle is directly related to its radius. The circumference is the distance around the circle, while the radius is the distance from the center to any point on the circle. The formula for finding the circumference from the radius is C = 2πr, and for finding the radius from the circumference is r = C / (2π).

How can the circumference be converted to radius?

To convert the circumference to radius, you can use the formula r = C / (2π), where r is the radius, C is the circumference, and π is the mathematical constant pi (approximately 3.14159).

What is the formula for finding the radius from the circumference?

The formula for finding the radius from the circumference is r = C / (2π), where r is the radius, C is the circumference, and π is the mathematical constant pi.

How is the radius related to the circumference in a circle?

In a circle, the radius is half the length of the diameter, and the circumference is the distance around the circle. The relationship between the two can be expressed using the formula C = 2πr, where C is the circumference and r is the radius.

Can the radius be used to calculate the circumference?

Yes, the radius can be used to calculate the circumference using the formula C = 2πr, where C is the circumference, π is the mathematical constant pi, and r is the radius.

Like? Share it with your friends

How to Find Radius from Circumference – Conversion and Formula

Table of Contents