Understanding Supplementary Angles
Table of Contents
Introduction
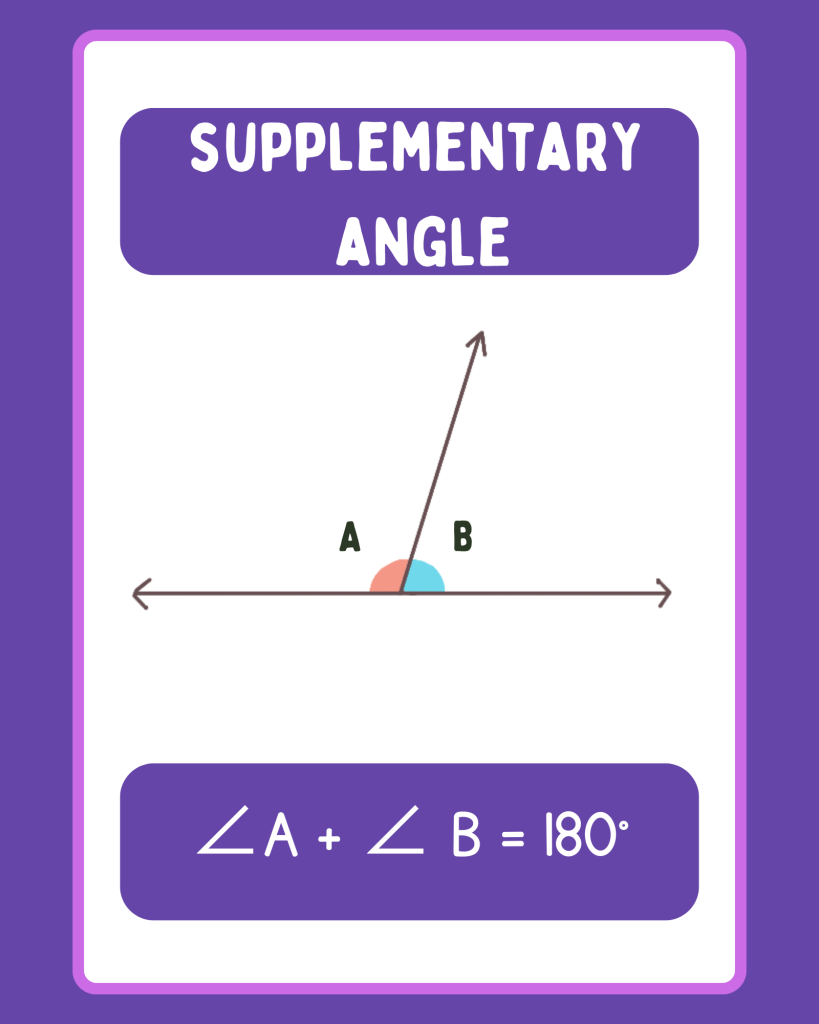
Supplementary Angles
Supplementary angles are a fundamental concept in geometry, playing a crucial role in understanding the relationship between angles and their measurements. Let’s delve into the realm of supplementary angles and explore their significance in geometric calculations and real-world scenarios.
Analogy of Definition
What are Supplementary Angles?
Supplementary angles refer to a pair of angles whose sum equals 180 degrees. This fundamental geometric concept plays a crucial role in various branches of mathematics, as well as in practical applications in fields such as engineering, architecture, and design.
When two supplementary angles are placed adjacent to each other, they share a common vertex and a common side, forming a straight line. This linear arrangement highlights the unique geometric relationship between the angles, as the straight line inherently measures 180 degrees. The property of forming a straight line when adjacent makes supplementary angles an essential element in constructing and analyzing geometric figures.
In mathematical applications, supplementary angles are often used to solve problems involving unknown angle measures. For instance, if one angle is known, the measure of its supplementary angle can be easily determined by subtracting the given angle from 180 degrees. This property is frequently utilized in solving equations and proving geometric theorems.
Method
Types of Supplementary Angles
Supplementary angles are two angles whose measures add up to 180 degrees. They can be classified into several types based on their positions and relationships with each other. Understanding these types helps in identifying and working with them in various geometric contexts. Here are the primary types of supplementary angles:
1. Adjacent Supplementary Angles
Adjacent supplementary angles share a common vertex and a common side, lying next to each other. They form a straight line, thus adding up to 180 degrees. These angles are also known as a linear pair. For example, if angle A measures 120 degrees and angle B measures 60 degrees, and they share a common side, they are adjacent supplementary angles.
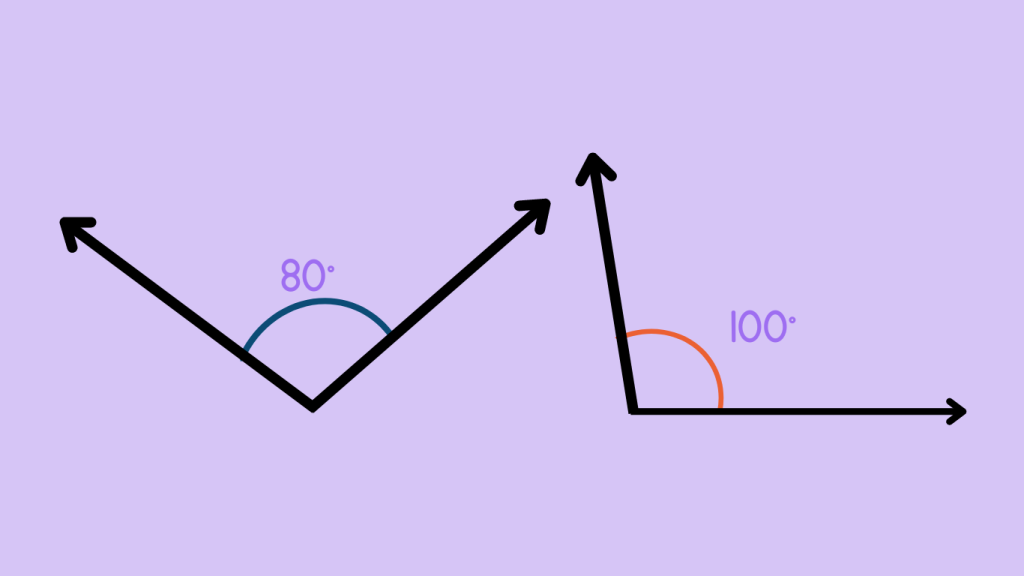
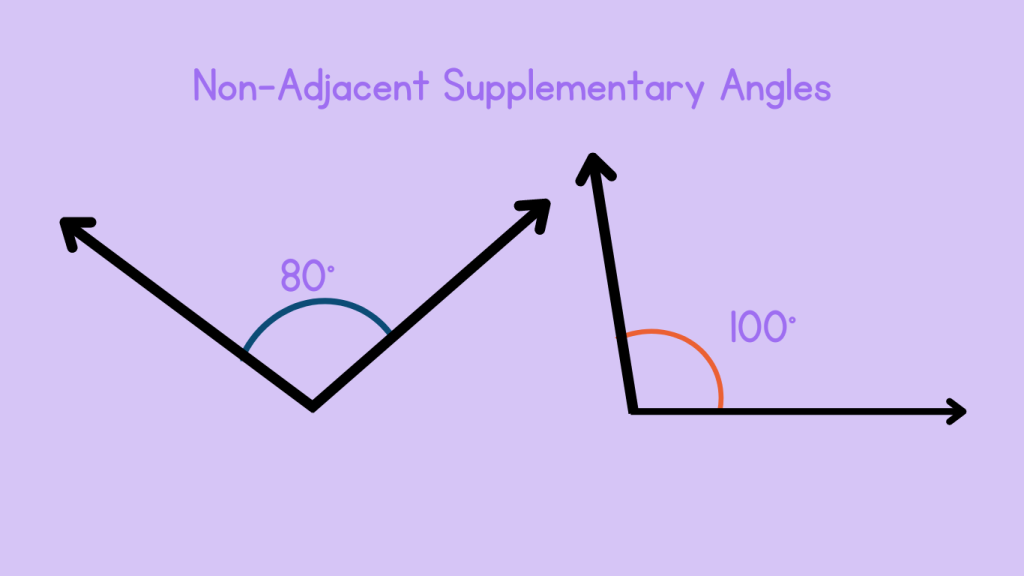
2. Non-Adjacent Supplementary Angles
Non-adjacent supplementary angles do not share a common vertex or side. They are separate from each other but their measures still sum to 180 degrees. These angles can be located anywhere in a plane as long as their total measure is 180 degrees. For instance, if angle C measures 110 degrees and angle D measures 70 degrees, they are non-adjacent supplementary angles.
Examples
Example 1:
Summary:
In this example, Angle 1 measures 120 degrees, and Angle 2 measures 60 degrees. By adding their measures, it is determined that the sum equals 180 degrees, confirming that Angle 1 and Angle 2 are indeed supplementary angles.
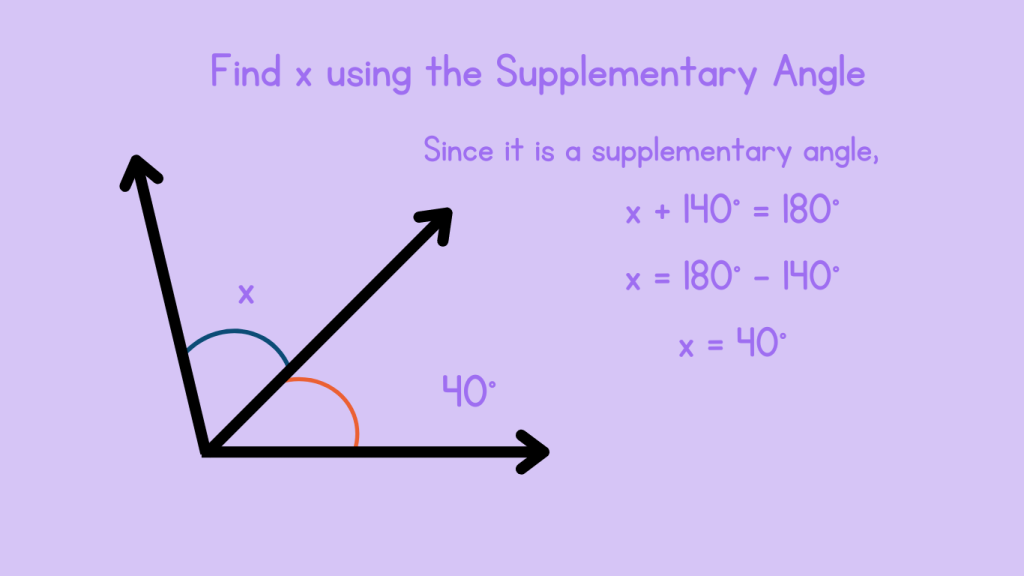
In this example, Angle 1 measures 120 degrees, and Angle 2 measures 60 degrees. By adding their measures, it is determined that the sum equals 180 degrees, confirming that Angle 1 and Angle 2 are indeed supplementary angles.
Quiz
Tips and Tricks
1. Identifying Supplementary Angles
Tip: Add the measures of the angles. If the sum equals 180 degrees, the angles are supplementary.
2. Real-Life Application
Tip: Notice that the hour and minute hands form a straight line, representing supplementary angles.
Real life application
Scenario 1: The Clock Hands
Observing the hands of a clock at 6:00, notice that the hour and minute hands form a straight line, representing supplementary angles.
Scenario 2: Street Intersections
When two streets intersect to form a straight line, the angles created are supplementary, aiding in urban planning and traffic flow management.
FAQ's
Supplementary angles are a pair of angles whose sum is 180 degrees. When placed adjacent to each other, they form a straight line.
Complementary angles add up to 90 degrees, while supplementary angles add up to 180 degrees. Complementary angles form a right angle when placed adjacent to each other, while supplementary angles form a straight line.
Yes, two obtuse angles can be supplementary if their sum equals 180 degrees. For example, two angles measuring 100 degrees and 80 degrees are supplementary.
Yes, real-life examples of supplementary angles include the hands of a clock at 3:00, 6:00, 9:00, and 12:00, as well as the intersection of two streets forming a straight line.
In geometry, supplementary angles are utilized to solve problems involving the measurement of angles, the construction of shapes, and the analysis of intersecting lines and angles.
Like? Share it with your friends
Facebook
Twitter
LinkedIn